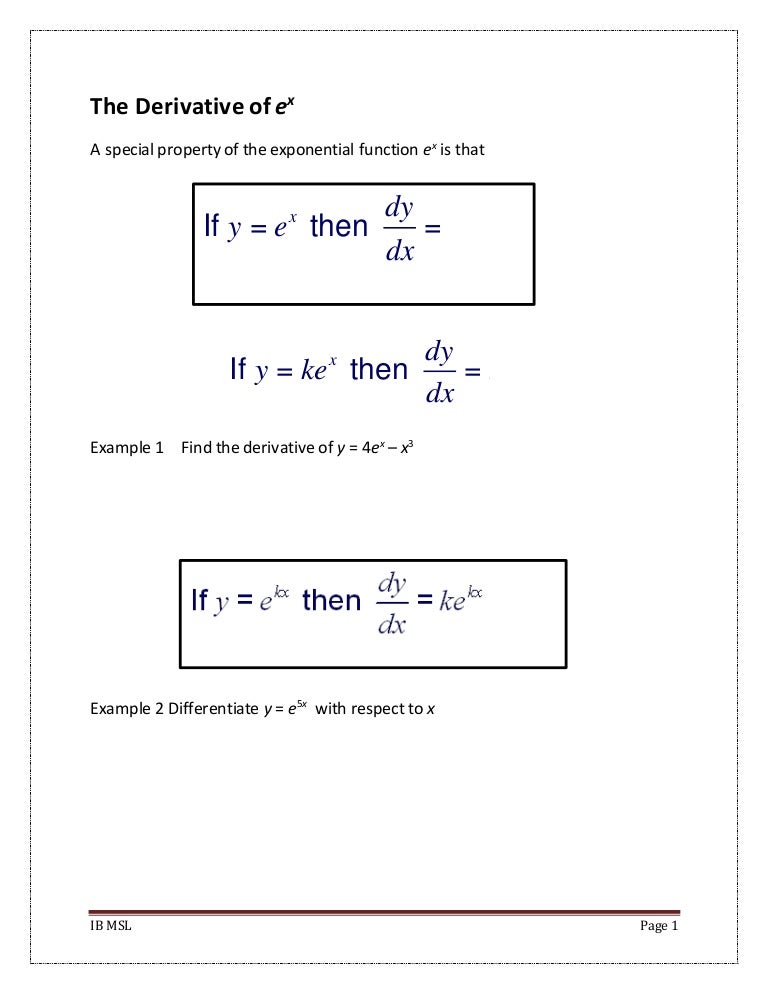
E X Derivative Proof

We only needed it here to prove the result above.

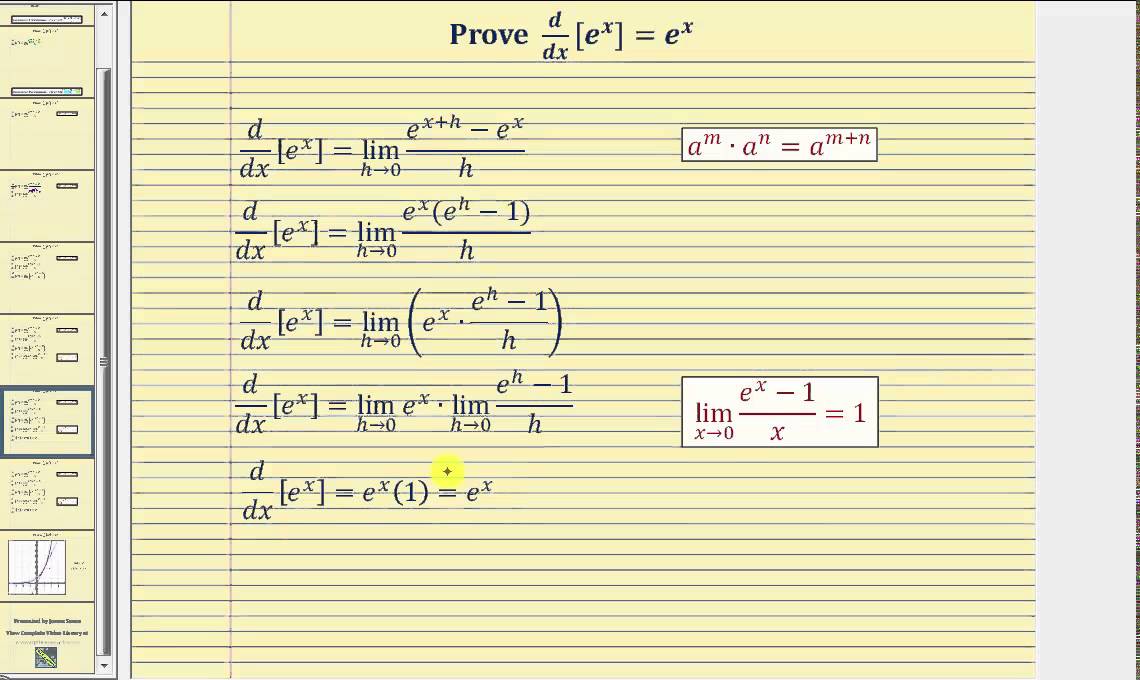
E x derivative proof. Related symbolab blog posts. Learn the proof for differentiation of exponential function e raised to power of x from first principle to prove d dx e x is equal to e x in calculus. Proof of derivative of e x the proof of the derivative of the natural exponential e x is presented using the limit definition of the derivative. Stack exchange network consists of 176 q a communities including stack overflow the largest most trusted online community for developers to learn share their knowledge and build their careers.

Derivative of e nx can be derived from the fundamental formula d dx e x e x in the above formula the internal derivative of power x with respect to x is 1 so the derivative of e x is e x. This website uses cookies to ensure you get the best experience. The derivative of e x is found by applying the chain rule of derivatives and the knowledge that the derivative of e x is always e x which can be found using a more complicated proof. Similarly for d dx e nx the e nx should be treated as e.

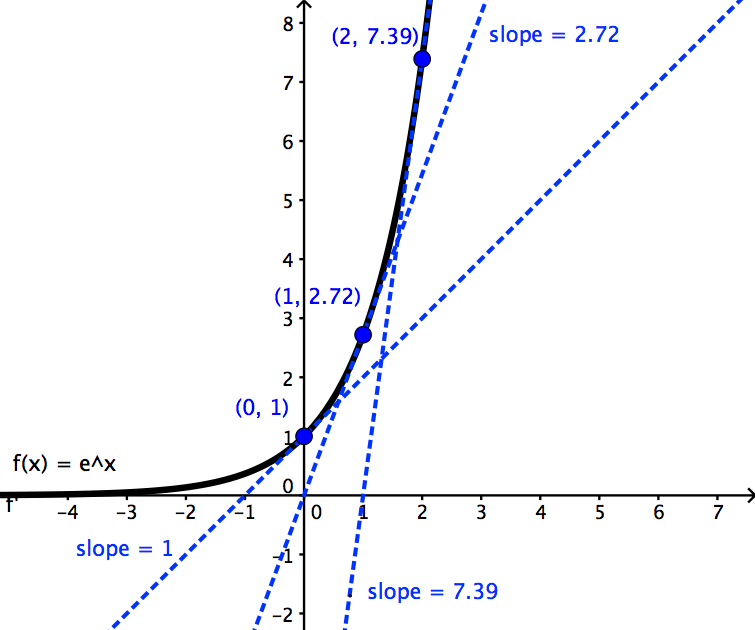
Proof that the derivative of e x is e x. The derivative of e x is e x. This means that for every x value the slope at that point is equal to the y value. We can now apply that to calculate the derivative of other functions involving the exponential.

Advertisement the chain rule of derivatives states that a composite function s derivative can be found by multiplying the inside function s derivative and the outside function s derivative. Watch the next lesson. Limit definition proof of e x. Proof that the derivative of e x is e x.

Derivative of e x proofs. This function is unusual because it is the exact same as its derivative. Type in any function derivative to get the solution steps and graph. This is one of the properties that makes the exponential function really important.

By laws of. Free derivative calculator differentiate functions with all the steps.